Qui aura 20 en maths ?

💯 Teste ton niveau de maths et tente de gagner un des lots !S'inscrire au jeu  →

Nouveau

🔥 Découvre nos fiches d'exercices gratuites avec corrections en vidéo !Accéder aux fiches  →

Matrices et transformations géométriques dans le plan : La symétrie axiale par rapport à l'axe des ordonnées - Exercice 3

3 min
5
Question 1

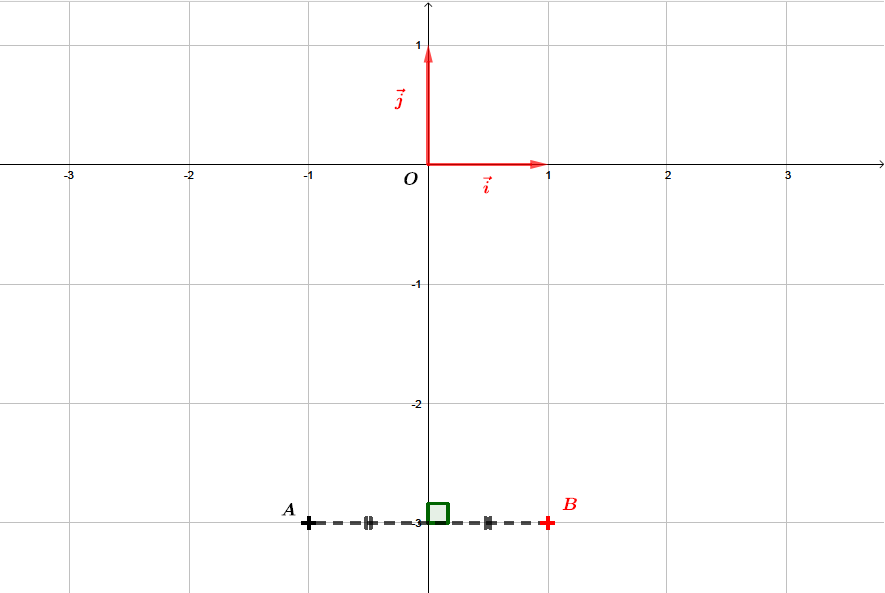
Dans un repère orthonormé direct (O;i→;j→)\left(O;\overrightarrow{i} ;\overrightarrow{j} \right), on donne le point A(−1;−3)A\left(-1;-3\right) .
Déterminer les coordonnées du point BB image du point AA par la symétrie axiale par rapport à l'axe des ordonnées.

Correction
On se place dans un repère orthonormé direct (O;i→;j→)\left(O;\overrightarrow{i} ;\overrightarrow{j} \right) du plan.
  • Soient A(xA;yA)A\left(x_{A}; y_{A}\right) et B(xB;yB)B\left(x_{B}; y_{B}\right) deux points du plan .
  • BB est l'image de AA par symeˊtrie axiale par rapport aˋ l’axe des ordonneˊes\red{\text{symétrie axiale par rapport à l'axe des ordonnées}} si et seulement si (xByB)=(−1001)×(xAyA)\left(\begin{array}{c} {x_{B} } \\ {y_{B} } \end{array}\right)=\left(\begin{array}{cc} {-1} & {0} \\ {0} & {1} \end{array}\right)\times \left(\begin{array}{c} {x_{A} } \\ {y_{A} } \end{array}\right)
    • Il nous suffit d'appliquer la formule du rappel. Il vient alors que :
    (xByB)=(−1001)×(xAyA)\left(\begin{array}{c} {x_{B} } \\ {y_{B} } \end{array}\right)=\left(\begin{array}{cc} {-1} & {0} \\ {0} & {1} \end{array}\right)\times \left(\begin{array}{c} {x_{A} } \\ {y_{A} } \end{array}\right)
    (xByB)=(−1001)×(−1−3)\left(\begin{array}{c} {x_{B} } \\ {y_{B} } \end{array}\right)=\left(\begin{array}{cc} {{\color{blue}{-1}}} & {{\color{blue}{0}}} \\ {{\color{red}{0}}} & {{\color{red}{1}}} \end{array}\right)\times \left(\begin{array}{c} {{\color{green}{-1}} } \\ {{\color{green}{-3}} } \end{array}\right)
    (xByB)=((−1)×(−1)+0×(−3)0×(−1)+1×(−3))\left(\begin{array}{c} {x_{B} } \\ {y_{B} } \end{array}\right)=\left(\begin{array}{c} {\left({\color{blue}{-1}}\right)\times {\color{green}{\left(-1\right)}}+{\color{blue}{0}}\times {\color{green}{\left(-3\right)}}} \\ {{\color{red}{0}}\times \color{green}{\left(-1\right)}+{\color{red}{1}}\times \left(-3\right)} \end{array}\right)
    (xByB)=(1+00−3)\left(\begin{array}{c} {x_{B} } \\ {y_{B} } \end{array}\right)=\left(\begin{array}{c} {1+0} \\ {0-3} \end{array}\right)
    Ainsi :
    (xByB)=(1−3)\left(\begin{array}{c} {x_{B} } \\ {y_{B} } \end{array}\right)=\left(\begin{array}{c} {1} \\ {-3} \end{array}\right)

    Les coordonnées du point BB image du point AA par la symétrie axiale par rapport à l'axe des ordonnées sont B(1;−3)B\left(1;-3\right)