Limites de fonctions

Lire des limites à l'aide d'une représentation graphique - Exercice 2

3 min
5
Question 1

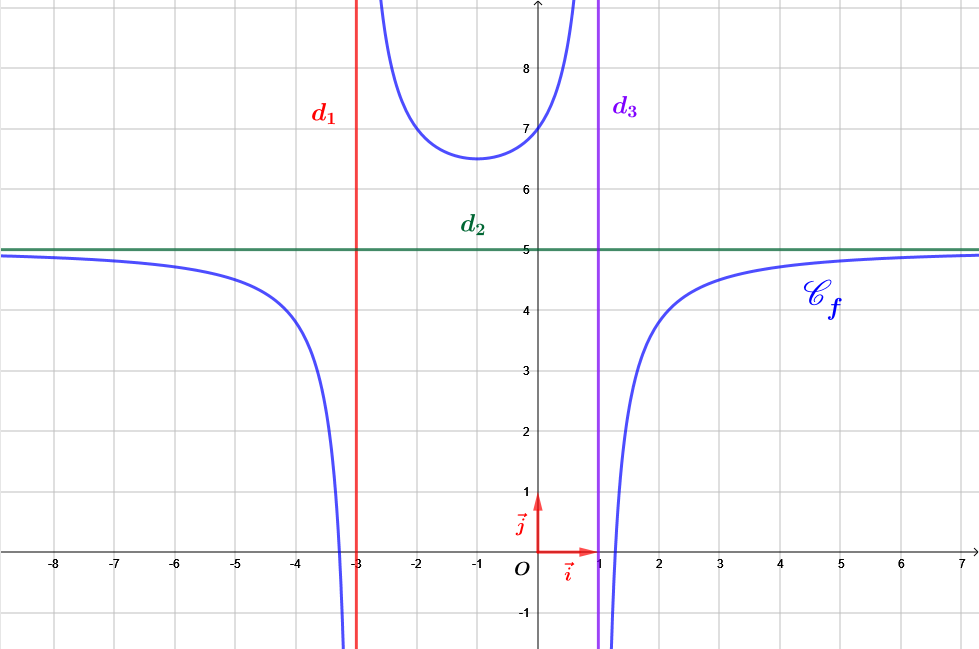
Par lecture graphique, conjecturer les limites de la fonction ff .

Correction
Si lim⁡x→+∞f(x)=l\lim\limits_{x\to +\infty } f(x) =l où ll est une valeur finie alors la fonction ff admet une asymptote horizontale d'équation y=ly=l
Si lim⁡x→−∞f(x)=l\lim\limits_{x\to -\infty } f(x) =l où ll est une valeur finie alors la fonction ff admet une asymptote horizontale d'équation y=ly=l
D'après la représentation graphique , on lit :
lim⁡x→−∞f(x)=5\lim\limits_{x\to -\infty } f\left(x\right) =5 et lim⁡x→+∞f(x)=5\lim\limits_{x\to +\infty } f\left(x\right) =5
Comme lim⁡x→−∞f(x)=5\lim\limits_{x\to -\infty } f\left(x\right) =5 alors la fonction ff admet une asymptote horizontale d'équation y=5y=5
Comme lim⁡x→+∞f(x)=5\lim\limits_{x\to +\infty } f\left(x\right) =5 alors la fonction ff admet une asymptote horizontale d'équation y=5y=5
Si lim⁡x→nombref(x)=+∞\lim\limits_{x\to \text{nombre}} f(x) =+\infty alors la fonction ff admet une asymptote verticale d'équation x=nombrex=\text{nombre}
Si lim⁡x→nombref(x)=−∞\lim\limits_{x\to \text{nombre}} f(x) =-\infty alors la fonction ff admet une asymptote verticale d'équation x=nombrex=\text{nombre}
Ainsi :
lim⁡x→−3−f(x)=−∞\lim\limits_{x\to -3^{-} } f\left(x\right)=-\infty alors la fonction ff admet une asymptote verticale d'équation x=−3x=-3
lim⁡x→−3+f(x)=+∞\lim\limits_{x\to -3^{+} } f\left(x\right)=+\infty alors la fonction ff admet une asymptote verticale d'équation x=−3x=-3
lim⁡x→1−f(x)=+∞\lim\limits_{x\to 1^{-} } f\left(x\right)=+\infty alors la fonction ff admet une asymptote verticale d'équation x=1x=1
lim⁡x→1+f(x)=−∞\lim\limits_{x\to 1^{+} } f\left(x\right)=-\infty alors la fonction ff admet une asymptote verticale d'équation x=1x=1