Qui aura 20 en maths ?
💯 Teste ton niveau de maths et tente de gagner un des lots !S'inscrire au jeu →
Nouveau
🔥 Découvre nos fiches d'exercices gratuites avec corrections en vidéo !Accéder aux fiches →
Etude de fonctions avec les exponentielles - Exercice 1
25 min
40
Question 1
Soit la fonction définie sur par
Etudier les variations de .
Correction
Soit
est dérivable sur
Ici on reconnaît la forme avec et .
On note
Ainsi : et , enfin
Il vient alors que :
. Pensez à factoriser par .
Ainsi :
Pour tout réel , on a et .
De plus :
On en déduit le tableau de variation suivant :
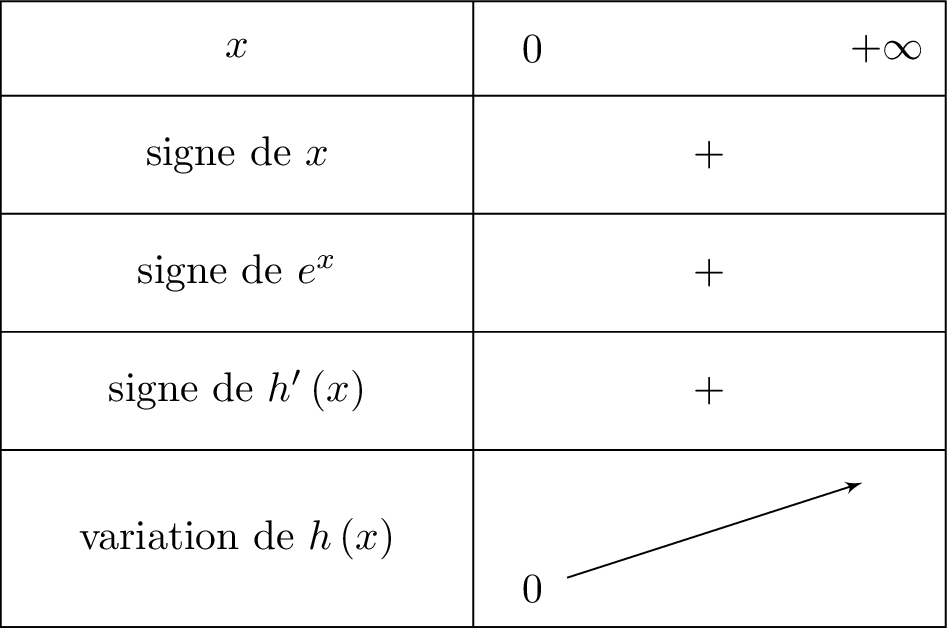
est dérivable sur
Ici on reconnaît la forme avec et .
On note
Ainsi : et , enfin
Il vient alors que :
. Pensez à factoriser par .
Ainsi :
Pour tout réel , on a et .
De plus :
On en déduit le tableau de variation suivant :

Question 2
En déduire le signe de sur l'intervalle .
Correction
D'après le tableau de variation obtenue à la question :
 On vérifie aisément que le minimum de vaut lorsque .
On vérifie aisément que le minimum de vaut lorsque .
Cela signifie donc que , pour tout réel , on a : .

Cela signifie donc que , pour tout réel , on a : .
Question 3
Soit la fonction définie sur par
Déterminer les limites de aux bornes de son ensemble de définition.
Correction
Finalement :
Question 4
Dresser le tableau de variation complet de la fonction .
Correction
Soit
est dérivable sur
Ici on reconnaît la forme avec et .
Ainsi et .
Il vient alors que
Pour tout réel , on vérifie aisément que et d'après la question nous savons que . On en déduit le tableau de variation de , ci dessous :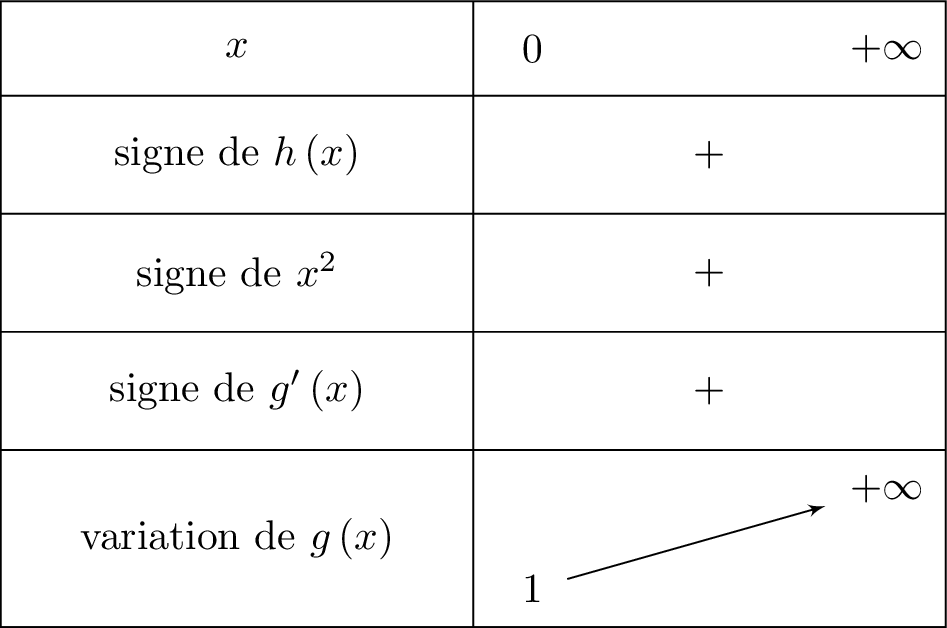
est dérivable sur
Ici on reconnaît la forme avec et .
Ainsi et .
Il vient alors que
Pour tout réel , on vérifie aisément que et d'après la question nous savons que . On en déduit le tableau de variation de , ci dessous :
