Qui aura 20 en maths ?

💯 Teste ton niveau de maths et tente de gagner un des lots !S'inscrire au jeu  →

Nouveau

🔥 Découvre nos fiches d'exercices gratuites avec corrections en vidéo !Accéder aux fiches  →

Savoir lire des coordonnées d'un vecteur dans un repère - Exercice 3

5 min
10
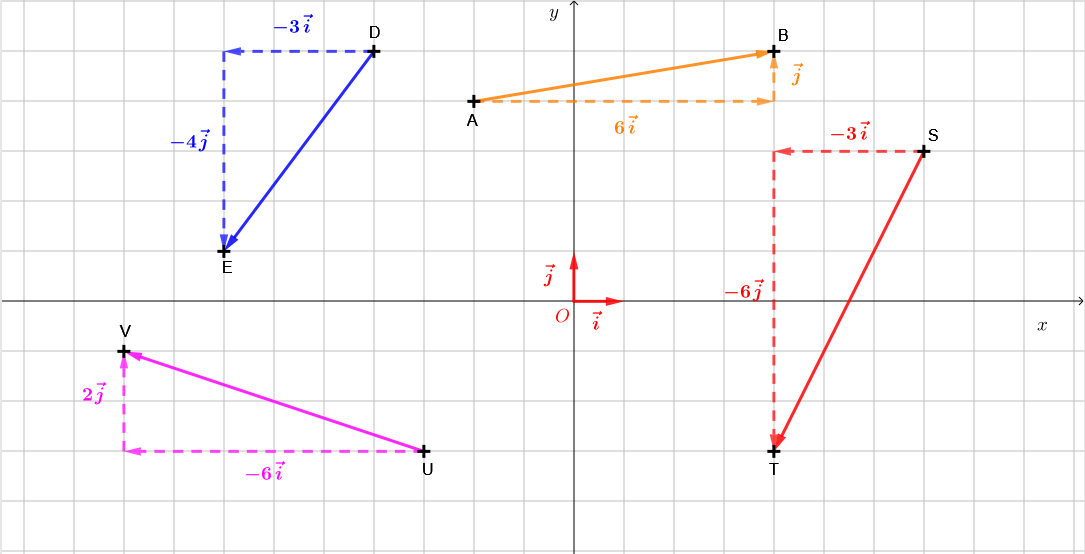
On considère le plan muni d'un repère (0;i→;j→)\left(0;\overrightarrow{i} ;\overrightarrow{j} \right) ci-dessous .
Question 1

Lire les coordonnées des vecteurs DE→\overrightarrow{DE} ; ST→\overrightarrow{ST} ; UV→\overrightarrow{UV} et AB→\overrightarrow{AB} .

Correction
Sur la figure, nous pouvons lire que :
  • ST→=−3i⃗−6j⃗\overrightarrow{ST}=-3\vec{i}-6\vec{j} donc ST→(−3−6)\overrightarrow{ST} \left(\begin{array}{c} {-3} \\ {-6} \end{array}\right)
  • DE→=−3i⃗−4j⃗\overrightarrow{DE}=-3\vec{i}-4\vec{j} donc DE→(−3−4)\overrightarrow{DE} \left(\begin{array}{c} {-3} \\ {-4} \end{array}\right)
  • UV→=−6i⃗+2j⃗\overrightarrow{UV}=-6\vec{i}+2\vec{j} donc UV→(−62)\overrightarrow{UV} \left(\begin{array}{c} {-6} \\ {2} \end{array}\right)
  • AB→=6i⃗+1j⃗\overrightarrow{AB}=6\vec{i}+1\vec{j} donc AB→(61)\overrightarrow{AB} \left(\begin{array}{c} {6} \\ {1} \end{array}\right)