Qui aura 20 en maths ?
💯 Le grand concours 100% Terminale revient le 31 janvier 2026 à l'ESIEA Paris !Découvrir →
Nouveau
🔥 Découvre nos fiches d'exercices gratuites avec corrections en vidéo !Accéder aux fiches →
Pour le plaisir - Exercice 1
20 min
40
Un exercice pour vérifier si l'on maîtrise l'art de la limite.
Déterminer la limite ci-dessous :
Déterminer la limite ci-dessous :
Question 1
Correction
La fonction est dérivable sur , en tant que composée de fonction elles mêmes dérivables sur .
Puis, la fonction est également dérivable sur , en tant que composée de fonction elles mêmes dérivables sur .
De plus on a la dérivée du dénominateur qui est donnée par :
Donc, pris en , on trouve que :
En outre, on constate que :
Nous avons donc une limite à déterminer qui se trouve être une forme indéterminée du type . Nous allons donc appliquer la règle de l'. On a donc :
Dans ce cas, ce dernier résultat implique que la limite recherchée vaut :
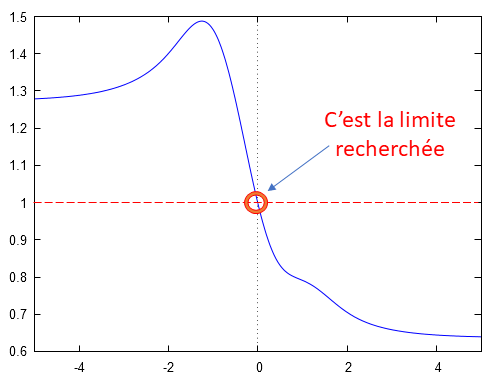
Graphiquement, on obtient :
Puis, la fonction est également dérivable sur , en tant que composée de fonction elles mêmes dérivables sur .
De plus on a la dérivée du dénominateur qui est donnée par :
Donc, pris en , on trouve que :
En outre, on constate que :
Nous avons donc une limite à déterminer qui se trouve être une forme indéterminée du type . Nous allons donc appliquer la règle de l'. On a donc :
Dans ce cas, ce dernier résultat implique que la limite recherchée vaut :
Graphiquement, on obtient :

Signaler une erreur
Aide-nous à améliorer nos contenus en signalant les erreurs ou problèmes que tu penses avoir trouvés.
Connecte-toi ou crée un compte pour signaler une erreur.