Qui aura 20 en maths ?

💯 Le grand concours 100% Terminale revient le 31 mai 2026 en ligne !Découvrir  →

Nouveau

🔥 Découvre nos fiches d'exercices gratuites avec corrections en vidéo !Accéder aux fiches  →

Calculs de dérivées et de différentielles : Mise en pratique épisode 6 - Exercice 1

1 h
90
La trigonométrie hyperbolique repose sur l'existence des trois fonctions suivantes :
∙  x∈R⟼cosh⁡(x)=ex+e−x2\bullet \,\, x \in \mathbb{R} \longmapsto \cosh(x) = \dfrac{e^x + e^{-x}}{2}
∙  x∈R⟼sinh⁡(x)=ex−e−x2\bullet \,\, x \in \mathbb{R} \longmapsto \sinh(x) = \dfrac{e^x - e^{-x}}{2}
∙  x∈R⟼tanh⁡(x)=sinh⁡(x)cosh⁡(x)=ex−e−xex+e−x\bullet \,\, x \in \mathbb{R} \longmapsto \tanh(x) = \dfrac{\sinh(x)}{\cosh(x)} = \dfrac{e^x - e^{-x}}{e^x + e^{-x}}
En terme de dérivation, on a les résultats suivants :
∙  x∈R⟼cosh⁡′(x)=sinh⁡(x)\bullet \,\, x \in \mathbb{R} \longmapsto \cosh'(x) = \sinh(x)
∙  x∈R⟼sinh⁡′(x)=cosh⁡(x)\bullet \,\, x \in \mathbb{R} \longmapsto \sinh'(x) = \cosh(x)
∙  x∈R⟼tanh⁡′(x)=1cosh⁡2(x)=1−tanh⁡2(x)\bullet \,\, x \in \mathbb{R} \longmapsto \tanh'(x) = \dfrac{1}{\cosh^2(x)} = 1 - \tanh^2(x)
La relation principale de la trigonométrie hyperbolique est :
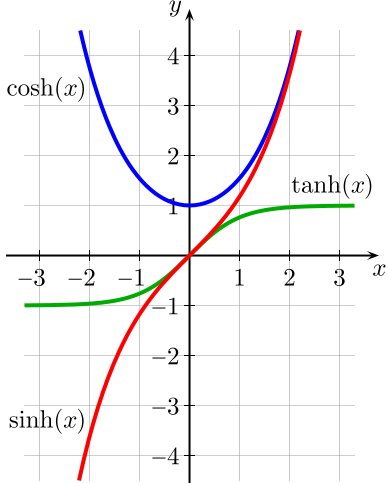
∀x∈R,  cosh⁡2(x)−sinh⁡2(x)=1{\color{red}{\boxed{\forall x \in \mathbb{R}, \,\, \cosh^2(x) - \sinh^2(x) = 1}}}
Il est important de bien avoir en "tête" les trois graphes représentatifs de ces fonctions :

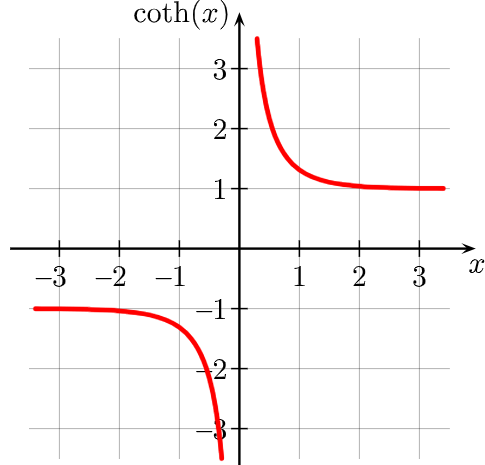
Rajoutons qu'il existe la cotangente hyperbolique, notée cotanh\mathrm{cotanh} (ou aussi coth⁡)\coth), et qui est définie comme :
∙  x∈R⋆⟼cotanh(x)=cosh⁡(x)sinh⁡(x)=1tanh⁡(x)=ex+e−xex−e−x\bullet \,\, x \in \mathbb{R}^\star \longmapsto \mathrm{cotanh}(x) = \dfrac{\cosh(x)}{\sinh(x)} = \dfrac{1}{\tanh(x)} = \dfrac{e^x + e^{-x}}{e^x - e^{-x}}
∙  x∈R⋆⟼cotanh′(x)=−1sinh⁡2(x)=1−cotanh2(x)\bullet \,\, x \in \mathbb{R}^\star \longmapsto \mathrm{cotanh}'(x) = -\dfrac{1}{\sinh^2(x)} = 1 -\mathrm{cotanh}^2(x)
Son graphe représentatif est :
Pour chacune des fonctions ff qui vous sont proposées dans cet exercice, déterminer la fonction dérivée f′f' et la différentielle associée dfdf.
Question 1

Soit x∈  [0 ; 2]⟼f(x)=cosh⁡(xsinh⁡(ex))x \in \,\,[0\,;\,2] \longmapsto f(x) = \cosh\left( \dfrac{x}{\sinh(e^x)}\right)

Correction
On a :
f′(x)=(cosh⁡(xsinh⁡(ex)))′=(xsinh⁡(ex))′×sinh⁡(xsinh⁡(ex))f'(x) = \left(\cosh\left( \dfrac{x}{\sinh(e^x)}\right)\right)' = \left( \dfrac{x}{\sinh(e^x)}\right)' \times \sinh\left( \dfrac{x}{\sinh(e^x)}\right)
Soit :
f′(x)=(x′sinh⁡(ex)−x(sinh⁡(ex))′sinh⁡2(ex))×sinh⁡(xsinh⁡(ex))f'(x) = \left( \dfrac{x'\sinh(e^x) - x \left( \sinh(e^x)\right)'}{\sinh^2(e^x)}\right) \times \sinh\left( \dfrac{x}{\sinh(e^x)}\right)
Soit encore :
f′(x)=(sinh⁡(ex)−x(ex)′sinh⁡′(ex)sinh⁡2(ex))×sinh⁡(xsinh⁡(ex))f'(x) = \left( \dfrac{\sinh(e^x) - x \left(e^x\right)'\sinh'(e^x)}{\sinh^2(e^x)}\right) \times \sinh\left( \dfrac{x}{\sinh(e^x)}\right)
Ce qui nous donne :
f′(x)=(sinh⁡(ex)−xexcosh⁡(ex)sinh⁡2(ex))×sinh⁡(xsinh⁡(ex))f'(x) = \left( \dfrac{\sinh(e^x) - x e^x \cosh(e^x)}{\sinh^2(e^x)}\right) \times \sinh\left( \dfrac{x}{\sinh(e^x)}\right)
Ainsi :
f′(x)=(sinh⁡(ex)sinh⁡2(ex)−xexcosh⁡(ex)sinh⁡2(ex))×sinh⁡(xsinh⁡(ex))f'(x) = \left( \dfrac{\sinh(e^x)}{\sinh^2(e^x)} - \dfrac{x e^x \cosh(e^x)}{\sinh^2(e^x)}\right) \times \sinh\left( \dfrac{x}{\sinh(e^x)}\right)
Comme sinh⁡(ex)≠0\sinh(e^x) \neq 0, on en déduit que :
f′(x)=(1sinh⁡(ex)−xexcosh⁡(ex)sinh⁡2(ex))×sinh⁡(xsinh⁡(ex))f'(x) = \left( \dfrac{1}{\sinh(e^x)} - \dfrac{x e^x \cosh(e^x)}{\sinh^2(e^x)}\right) \times \sinh\left( \dfrac{x}{\sinh(e^x)}\right)
Ainsi :
f′(x)=1sinh⁡(ex)(1−xexcosh⁡(ex)sinh⁡(ex))×sinh⁡(xsinh⁡(ex))f'(x) = \dfrac{1}{\sinh(e^x)} \left( 1 - \dfrac{x e^x \cosh(e^x)}{\sinh(e^x)}\right) \times \sinh\left( \dfrac{x}{\sinh(e^x)}\right)
Ou encore :
f′(x)=1sinh⁡(ex)(1−xexcosh⁡(ex)sinh⁡(ex))×sinh⁡(xsinh⁡(ex))f'(x) = \dfrac{1}{\sinh(e^x)} \left( 1 - x e^x \dfrac{\cosh(e^x)}{\sinh(e^x)}\right) \times \sinh\left( \dfrac{x}{\sinh(e^x)}\right)
Or ex≠0e^x \neq 0, on en déduit que sinh⁡(ex)≠0\sinh(e^x) \neq 0 et ainsi cosh⁡(ex)sinh⁡(ex)=cotanh(ex)\dfrac{\cosh(e^x)}{\sinh(e^x)} = \mathrm{cotanh}(e^x) existe bien. Et de fait :
f′(x)=1sinh⁡(ex)(1−xexcotanh(ex))×sinh⁡(xsinh⁡(ex))f'(x) = \dfrac{1}{\sinh(e^x)} \left( 1 - x e^x \mathrm{cotanh}(e^x)\right) \times \sinh\left( \dfrac{x}{\sinh(e^x)}\right)
Finalement :
f′(x)=(1−xexcotanh(ex))sinh⁡(xsinh⁡(ex))sinh⁡(ex){\color{red}{\boxed{f'(x) = \left( 1 - x e^x \mathrm{cotanh}(e^x)\right) \dfrac{\sinh\left( \dfrac{x}{\sinh(e^x)}\right)}{\sinh(e^x)} }}}
On a alors :
f′(x)=dfdx(x)f'(x) = \dfrac{df}{dx}(x)
En faisant usage de l'écriture de LeibnizLeibniz, on peut donc écrire que :
df(x)=f′(x) dxdf(x) = f'(x) \, dx
Ainsi, la différentielle de ff est donnée par l'expression suivante :
df(x)=(1−xexcotanh(ex))sinh⁡(xsinh⁡(ex))sinh⁡(ex) dx{\color{red}{\boxed{df(x) = \left( 1 - x e^x \mathrm{cotanh}(e^x)\right) \dfrac{\sinh\left( \dfrac{x}{\sinh(e^x)}\right)}{\sinh(e^x)} \, dx}}}
Question 2

Soit x∈  ]0 ; 1]⟼f(x)=(sinh⁡(xcosh⁡(x)))2x \in \,\,]0\,;\,1] \longmapsto f(x) = \left(\sinh\left( x^{\cosh(x)}\right)\right)^2

Correction
On a :
f′(x)=((sinh⁡(xcosh⁡(x)))2)′=2sinh⁡(xcosh⁡(x))(sinh⁡(xcosh⁡(x)))′f'(x) = \left(\left(\sinh\left( x^{\cosh(x)}\right)\right)^2\right)' = 2 \sinh\left( x^{\cosh(x)}\right) \left(\sinh\left( x^{\cosh(x)}\right)\right)'
Soit :
f′(x)=2sinh⁡(xcosh⁡(x))(xcosh⁡(x))′sinh⁡′(xcosh⁡(x))f'(x) = 2 \sinh\left( x^{\cosh(x)}\right) \left(x^{\cosh(x)}\right)'\sinh'\left( x^{\cosh(x)}\right)
Donc :
f′(x)=2sinh⁡(xcosh⁡(x))(eln⁡(xcosh⁡(x)))′cosh⁡(xcosh⁡(x))f'(x) = 2 \sinh\left( x^{\cosh(x)}\right) \left(e^{\ln \left(x^{\cosh(x)}\right)}\right)'\cosh\left( x^{\cosh(x)}\right)
Ce qui nous donne (puisque x>0x > 0) :
f′(x)=2sinh⁡(xcosh⁡(x))(ecosh⁡(x)ln⁡(x))′cosh⁡(xcosh⁡(x))f'(x) = 2 \sinh\left( x^{\cosh(x)}\right) \left(e^{\cosh(x)\ln(x)}\right)'\cosh\left( x^{\cosh(x)}\right)
Ainsi, on obtient :
f′(x)=2sinh⁡(xcosh⁡(x))(cosh⁡(x)ln⁡(x))′ecosh⁡(x)ln⁡(x)cosh⁡(xcosh⁡(x))f'(x) = 2 \sinh\left( x^{\cosh(x)}\right) \left(\cosh(x)\ln(x)\right)'e^{\cosh(x)\ln(x)}\cosh\left( x^{\cosh(x)}\right)
On peut donc écrire que :
f′(x)=2sinh⁡(xcosh⁡(x))(sinh⁡(x)ln⁡(x)+cosh⁡(x)x)ecosh⁡(x)ln⁡(x)cosh⁡(xcosh⁡(x))f'(x) = 2 \sinh\left( x^{\cosh(x)}\right) \left(\sinh(x)\ln(x) + \dfrac{\cosh(x)}{x}\right) e^{\cosh(x)\ln(x)}\cosh\left( x^{\cosh(x)}\right)
On peut écrire ceci sous la forme suivante :
f′(x)=2sinh⁡(xcosh⁡(x))(xsinh⁡(x)ln⁡(x)x+cosh⁡(x)x)ecosh⁡(x)ln⁡(x)cosh⁡(xcosh⁡(x))f'(x) = 2 \sinh\left( x^{\cosh(x)}\right) \left(\dfrac{x\sinh(x)\ln(x)}{x} + \dfrac{\cosh(x)}{x}\right) e^{\cosh(x)\ln(x)}\cosh\left( x^{\cosh(x)}\right)
En factorisant par sinh⁡(x)x\dfrac{\sinh(x)}{x} on trouve que :
f′(x)=2sinh⁡(x)xsinh⁡(xcosh⁡(x))(xln⁡(x)+cosh⁡(x)sinh⁡(x))ecosh⁡(x)ln⁡(x)cosh⁡(xcosh⁡(x))f'(x) = 2 \dfrac{\sinh(x)}{x}\sinh\left( x^{\cosh(x)}\right) \left(x\ln(x) + \dfrac{\cosh(x)}{\sinh(x)}\right) e^{\cosh(x)\ln(x)}\cosh\left( x^{\cosh(x)}\right)
Ce qui nous donne :
f′(x)=2sinh⁡(x)xsinh⁡(xcosh⁡(x))(xln⁡(x)+cotanh(x))ecosh⁡(x)ln⁡(x)cosh⁡(xcosh⁡(x))f'(x) = 2 \dfrac{\sinh(x)}{x}\sinh\left( x^{\cosh(x)}\right) \left(x\ln(x) + \mathrm{cotanh}(x)\right) e^{\cosh(x)\ln(x)}\cosh\left( x^{\cosh(x)}\right)
Puis, en remarquant que ecosh⁡(x)ln⁡(x)=xcosh⁡(x)e^{\cosh(x)\ln(x)} = x^{\cosh(x)}, on obtient :
f′(x)=2sinh⁡(x)xsinh⁡(xcosh⁡(x))(xln⁡(x)+cotanh(x))xcosh⁡(x)cosh⁡(xcosh⁡(x))f'(x) = 2 \dfrac{\sinh(x)}{x}\sinh\left( x^{\cosh(x)}\right) \left(x\ln(x) + \mathrm{cotanh}(x)\right) x^{\cosh(x)}\cosh\left( x^{\cosh(x)}\right)
De plus, on a xcosh⁡(x)x=xcosh⁡(x)−1\dfrac{x^{\cosh(x)}}{x} = x^{\cosh(x)-1}. Ce qui nous permet d'écrire que :
f′(x)=2sinh⁡(x)sinh⁡(xcosh⁡(x))(xln⁡(x)+cotanh(x))xcosh⁡(x)−1cosh⁡(xcosh⁡(x))f'(x) = 2 \sinh(x)\sinh\left( x^{\cosh(x)}\right) \left(x\ln(x) + \mathrm{cotanh}(x)\right) x^{\cosh(x)-1}\cosh\left( x^{\cosh(x)}\right)
Ecrivons cela sous la forme :
f′(x)=2sinh⁡(xcosh⁡(x))cosh⁡(xcosh⁡(x))sinh⁡(x)(xln⁡(x)+cotanh(x))xcosh⁡(x)−1f'(x) = 2 \sinh\left( x^{\cosh(x)}\right) \cosh\left( x^{\cosh(x)}\right)\sinh(x) \left(x\ln(x) + \mathrm{cotanh}(x)\right) x^{\cosh(x)-1}
Mais, pour XX réel, on a 2sinh⁡(X)cosh⁡(X)=sinh⁡(2X)2 \sinh\left(X\right) \cosh\left(X\right) = \sinh(2X). Ainsi 2sinh⁡(xcosh⁡(x))cosh⁡(xcosh⁡(x))=sinh⁡(2xcosh⁡(x)) 2 \sinh\left( x^{\cosh(x)}\right) \cosh\left( x^{\cosh(x)}\right) = \sinh\left( 2x^{\cosh(x)}\right)
Finalement :
f′(x)=(ln⁡(xx)+cotanh(x))sinh⁡(x)sinh⁡(2xcosh⁡(x))xcosh⁡(x)−1{\color{red}{\boxed{f'(x) = \left(\ln(x^x) + \mathrm{cotanh}(x)\right) \sinh(x)\sinh\left( 2x^{\cosh(x)}\right) x^{\cosh(x)-1} }}}
On a alors :
f′(x)=dfdx(x)f'(x) = \dfrac{df}{dx}(x)
En faisant usage de l'écriture de LeibnizLeibniz, on peut donc écrire que :
df(x)=f′(x) dxdf(x) = f'(x) \, dx
Ainsi, la différentielle de ff est donnée par l'expression suivante :
df(x)=(ln⁡(xx)+cotanh(x))sinh⁡(x)sinh⁡(2xcosh⁡(x))xcosh⁡(x)−1 dx{\color{red}{\boxed{df(x) = \left(\ln(x^x) + \mathrm{cotanh}(x)\right) \sinh(x)\sinh\left( 2x^{\cosh(x)}\right) x^{\cosh(x)-1} \, dx}}}
Question 3

Soit x∈R  ⟼f(x)=tanh⁡(xtanh⁡(x))x \in \mathbb{R} \,\, \longmapsto f(x) = \tanh\left( x \tanh(x)\right)

Correction
On a :
f′(x)=(tanh⁡(xtanh⁡(x)))′=(xtanh⁡(x))′×1cosh⁡2(xtanh⁡(x))f'(x) = \left(\tanh\left( x \tanh(x)\right)\right)' = \left( x \tanh(x)\right)'\times \dfrac{1}{\cosh^2\left( x \tanh(x)\right)}
Soit :
f′(x)=(x′tanh⁡(x)+xtanh⁡′(x))×1cosh⁡2(xtanh⁡(x))f'(x) = \left( x' \tanh(x) + x\tanh'(x)\right)\times \dfrac{1}{\cosh^2\left( x \tanh(x)\right)}
Soit encore :
f′(x)=(1tanh⁡(x)+x1cosh⁡2(x))×1cosh⁡2(xtanh⁡(x))f'(x) = \left( 1 \tanh(x) + x\dfrac{1}{\cosh^2(x)}\right)\times \dfrac{1}{\cosh^2\left( x \tanh(x)\right)}
Ainsi, on trouve que :
f′(x)=(tanh⁡(x)+xcosh⁡2(x))×1cosh⁡2(xtanh⁡(x))f'(x) = \left( \tanh(x) + \dfrac{x}{\cosh^2(x)}\right)\times \dfrac{1}{\cosh^2\left( x \tanh(x)\right)}
On a alors :
f′(x)=(sinh⁡(x)cosh⁡(x)+xcosh⁡2(x))×1cosh⁡2(xtanh⁡(x))f'(x) = \left( \dfrac{\sinh(x)}{\cosh(x)} + \dfrac{x}{\cosh^2(x)}\right)\times \dfrac{1}{\cosh^2\left( x \tanh(x)\right)}
Où encore :
f′(x)=(2cosh⁡(x)sinh⁡(x)2cosh⁡2(x)+2x2cosh⁡2(x))×1cosh⁡2(xtanh⁡(x))f'(x) = \left( \dfrac{2\cosh(x)\sinh(x)}{2\cosh^2(x)} + \dfrac{2x}{2\cosh^2(x)}\right)\times \dfrac{1}{\cosh^2\left( x \tanh(x)\right)}
Ce qui nous donne :
f′(x)=(sinh⁡(2x)2cosh⁡2(x)+2x2cosh⁡2(x))×1cosh⁡2(xtanh⁡(x))f'(x) = \left( \dfrac{\sinh(2x)}{2\cosh^2(x)} + \dfrac{2x}{2\cosh^2(x)}\right)\times \dfrac{1}{\cosh^2\left( x \tanh(x)\right)}
En factorisant :
f′(x)=12cosh⁡2(x)(sinh⁡(2x)+2x)×1cosh⁡2(xtanh⁡(x))f'(x) = \dfrac{1}{2\cosh^2(x)}\left(\sinh(2x) + 2x\right)\times \dfrac{1}{\cosh^2\left( x \tanh(x)\right)}
Finalement :
f′(x)=2x+sinh⁡(2x)2cosh⁡2(x)cosh⁡2(xtanh⁡(x)){\color{red}{\boxed{f'(x) = \dfrac{2x + \sinh(2x)}{2\cosh^2(x)\cosh^2\left( x \tanh(x)\right)} }}}
On a alors :
f′(x)=dfdx(x)f'(x) = \dfrac{df}{dx}(x)
En faisant usage de l'écriture de LeibnizLeibniz, on peut donc écrire que :
df(x)=f′(x) dxdf(x) = f'(x) \, dx
Ainsi, la différentielle de ff est donnée par l'expression suivante :
df(x)=2x+sinh⁡(2x)2cosh⁡2(x)cosh⁡2(xtanh⁡(x)) dx{\color{red}{\boxed{df(x) = \dfrac{2x + \sinh(2x)}{2\cosh^2(x)\cosh^2\left( x \tanh(x)\right)} \, dx}}}
Question 4

Soit x∈R⋆  ⟼f(x)=tanh⁡(xcosh⁡(2x)xsinh⁡(3x))x \in \mathbb{R}^\star \,\, \longmapsto f(x) = \tanh\left( \dfrac{x^{\cosh(2x)}}{x^{\sinh(3x)}} \right)

Correction
On a :
f′(x)=(tanh⁡(xcosh⁡(2x)xsinh⁡(3x)))′f'(x) = \left(\tanh\left( \dfrac{x^{\cosh(2x)}}{x^{\sinh(3x)}} \right)\right)'
Soit :
f′(x)=(xcosh⁡(2x)xsinh⁡(3x))′×1cosh⁡2(xcosh⁡(2x)xsinh⁡(3x))f'(x) = \left(\dfrac{x^{\cosh(2x)}}{x^{\sinh(3x)}} \right)'\times \dfrac{1}{\cosh^2\left( \dfrac{x^{\cosh(2x)}}{x^{\sinh(3x)}}\right)}
Soit encore :
f′(x)=(xcosh⁡(2x)−sinh⁡(3x))′×1cosh⁡2(xcosh⁡(2x)−sinh⁡(3x))f'(x) = \left(x^{\cosh(2x)-\sinh(3x)} \right)'\times \dfrac{1}{\cosh^2\left( x^{\cosh(2x)-\sinh(3x)}\right)}
Avec :
(xcosh⁡(2x)−sinh⁡(3x))′=(eln⁡(xcosh⁡(2x)−sinh⁡(3x)))′=(e(cosh⁡(2x)−sinh⁡(3x))ln⁡(x))′ \left(x^{\cosh(2x)-\sinh(3x)} \right)' = \left(e^{\ln\left(x^{\cosh(2x)-\sinh(3x)}\right)} \right)' = \left(e^{\left( \cosh(2x)-\sinh(3x) \right)\ln(x)} \right)'
Soit encore :
(xcosh⁡(2x)−sinh⁡(3x))′=((cosh⁡(2x)−sinh⁡(3x))ln⁡(x))′e(cosh⁡(2x)−sinh⁡(3x))ln⁡(x) \left(x^{\cosh(2x)-\sinh(3x)} \right)' = \left(\left( \cosh(2x)-\sinh(3x) \right)\ln(x) \right)'e^{\left( \cosh(2x)-\sinh(3x) \right)\ln(x)}
Donc :
(xcosh⁡(2x)−sinh⁡(3x))′=((cosh⁡(2x)−sinh⁡(3x))ln⁡(x))′xcosh⁡(2x)−sinh⁡(3x) \left(x^{\cosh(2x)-\sinh(3x)} \right)' = \left(\left( \cosh(2x)-\sinh(3x) \right)\ln(x) \right)'x^{\cosh(2x)-\sinh(3x)}
Avec :
((cosh⁡(2x)−sinh⁡(3x))ln⁡(x))′=(2sinh⁡(2x)−3cosh⁡(3x))ln⁡(x)+(cosh⁡(2x)−sinh⁡(3x))1x\left(\left( \cosh(2x)-\sinh(3x) \right)\ln(x) \right)' = \left( 2\sinh(2x)-3\cosh(3x) \right)\ln(x) + \left( \cosh(2x)-\sinh(3x) \right)\dfrac{1}{x}
Soit encore :
((cosh⁡(2x)−sinh⁡(3x))ln⁡(x))′=(2xsinh⁡(2x)−3xcosh⁡(3x))ln⁡(x)+cosh⁡(2x)−sinh⁡(3x)x\left(\left( \cosh(2x)-\sinh(3x) \right)\ln(x) \right)' = \dfrac{\left( 2x\sinh(2x)-3x\cosh(3x) \right)\ln(x) + \cosh(2x)-\sinh(3x)}{x}
(xcosh⁡(2x)−sinh⁡(3x))′=(2xsinh⁡(2x)−3xcosh⁡(3x))ln⁡(x)+cosh⁡(2x)−sinh⁡(3x)xxcosh⁡(2x)−sinh⁡(3x) \left(x^{\cosh(2x)-\sinh(3x)} \right)' = \dfrac{\left( 2x\sinh(2x)-3x\cosh(3x) \right)\ln(x) + \cosh(2x)-\sinh(3x)}{x}x^{\cosh(2x)-\sinh(3x)}
Ce qui s'écrit également comme :
(xcosh⁡(2x)−sinh⁡(3x))′=((2xsinh⁡(2x)−3xcosh⁡(3x))ln⁡(x)+cosh⁡(2x)−sinh⁡(3x))xcosh⁡(2x)−sinh⁡(3x)−1 \left(x^{\cosh(2x)-\sinh(3x)} \right)' = \left(\left( 2x\sinh(2x)-3x\cosh(3x) \right)\ln(x) + \cosh(2x)-\sinh(3x) \right)x^{\cosh(2x)-\sinh(3x)-1}
Ceci nous permet d'obtenir l'expression de f′f' suivante :
f′(x)=((2xsinh⁡(2x)−3xcosh⁡(3x))ln⁡(x)+cosh⁡(2x)−sinh⁡(3x))xcosh⁡(2x)−sinh⁡(3x)−1cosh⁡2(xcosh⁡(2x)−sinh⁡(3x))f'(x) = \dfrac{\left(\left( 2x\sinh(2x)-3x\cosh(3x) \right)\ln(x) + \cosh(2x)-\sinh(3x) \right)x^{\cosh(2x)-\sinh(3x)-1}}{\cosh^2\left( x^{\cosh(2x)-\sinh(3x)}\right)}
Ou encore :
f′(x)=((2sinh⁡(2x)−3cosh⁡(3x))xln⁡(x)+cosh⁡(2x)−sinh⁡(3x))xcosh⁡(2x)−sinh⁡(3x)−1cosh⁡2(xcosh⁡(2x)−sinh⁡(3x))f'(x) = \dfrac{\left(\left( 2\sinh(2x)-3\cosh(3x) \right)x\ln(x) + \cosh(2x)-\sinh(3x) \right)x^{\cosh(2x)-\sinh(3x)-1}}{\cosh^2\left( x^{\cosh(2x)-\sinh(3x)}\right)}
Finalement :
f′(x)=((2sinh⁡(2x)−3cosh⁡(3x))ln⁡(xx)+cosh⁡(2x)−sinh⁡(3x))xcosh⁡(2x)−sinh⁡(3x)−1cosh⁡2(xcosh⁡(2x)−sinh⁡(3x)){\color{red}{\boxed{f'(x) = \dfrac{\left(\left( 2\sinh(2x)-3\cosh(3x) \right)\ln(x^x) + \cosh(2x)-\sinh(3x) \right)x^{\cosh(2x)-\sinh(3x)-1}}{\cosh^2\left( x^{\cosh(2x)-\sinh(3x)}\right)} }}}
On a alors :
f′(x)=dfdx(x)f'(x) = \dfrac{df}{dx}(x)
En faisant usage de l'écriture de LeibnizLeibniz, on peut donc écrire que :
df(x)=f′(x) dxdf(x) = f'(x) \, dx
Ainsi, la différentielle de ff est donnée par l'expression suivante :
df(x)=((2sinh⁡(2x)−3cosh⁡(3x))ln⁡(xx)+cosh⁡(2x)−sinh⁡(3x))xcosh⁡(2x)−sinh⁡(3x)−1cosh⁡2(xcosh⁡(2x)−sinh⁡(3x)) dx{\color{red}{\boxed{df(x) = \dfrac{\left(\left( 2\sinh(2x)-3\cosh(3x) \right)\ln(x^x) + \cosh(2x)-\sinh(3x) \right)x^{\cosh(2x)-\sinh(3x)-1}}{\cosh^2\left( x^{\cosh(2x)-\sinh(3x)}\right)} \, dx}}}

Signaler une erreur

Aide-nous à améliorer nos contenus en signalant les erreurs ou problèmes que tu penses avoir trouvés.

Connecte-toi ou crée un compte pour signaler une erreur.